Hydrogen, like all atoms, provides very distinctive lines when the frequency of its electromagnetic waves are measured as its electron changes between orbitals. These electromagnetic waves come in the form of packets, or photons, that are absorbed or emitted by an electron. Photons are absorbed if the electron takes energy to move away from the hydrogen nucleus and emitted if the electron moves closer to the hydrogen nucleus.
- Ionization Energy Of Hydrogen Atom
- Ionisation Energy Of Hydrogen Atom Is 13.6
- Ionization Energy Of Hydrogen Atom
- Ionisation Energy Of Hydrogen Formula
- Determining Ionization Energy
- Ionization Energy Of Hydrogen In Joules
WHAT AFFECTS IONISATION ENERGY? The value of the 1st Ionisation Energy depends on the electronic structure Hydrogen Helium Lithium The value for helium is higher than that for hydrogen because there are now two protons in the nucleus. The nuclear charge is greater so the pull on the outer electrons is larger. This is the energy per mole necessary to remove electrons from gaseous atoms or atomic ions. The first molar ionization energy applies to the neutral atoms. The second, third, etc., molar ionization energy applies to the further removal of an electron from a singly, doubly, etc., charged ion.
Despite the electron’s probability distribution in an atomic orbit, the energy transition states are very distinct when they move between orbitals. The photon energy and its wavelength are measured by spectral analysis such as hydrogen’s spectral lines below:
In wave theory, these transitions are described as a conversion from transverse to longitudinal wave energy and vice versa. Particles produce spherical, longitudinal waves that decrease with the square of the distance from the particle core. Two or more particles will produce waves that are amplified, their longitudinal wave amplitude is constructively or destructively added as a result of this interaction. Thus, this energy changes as the distance between the particles change. However, energy is always conserved. It transitions from one wave form to another.
Longitudinal wave energy is spherical, where each shell (n) in the wave equations is a longitudinal wavelength. Each spherical shell has an energy level that can be computed with the Longitudinal Energy equation. This energy can be transferred. Transverse waves are a secondary wave as a result of particle vibration. Whether it is particle annihilation or orbital transition, the electron will vibrate as it settles into its final position and this vibration creates a transverse wave. The difference in longitudinal energy is then transferred to vibrational energy in a transverse wave (photon). A photon will travel until reaching another particle, transferring its energy to longitudinal energy, once again affecting the wave amplitude between particles. The photon will be absorbed in this case and a particle once again moves to minimize its amplitude. It moves further from the atom’s core once the photon is absorbed.
Photons are transverse waves which we know as electromagnetic waves responsible for light, radio waves, microwaves and more. They are a result of short bursts of electron vibrations, creating a secondary transverse wave. Energy is conserved and we can calculate this as a difference in longitudinal energy between two or more particles.
Evidence
The evidence is an exact match for the hydrogen atom for both ionization and orbital transitions when using the wave equations to calculate photon energies and wavelengths. When an electron leaves the atom, or when the electron transitions orbitals, a photon is emitted or absorbed and this can be calculated using the wave equations. The calculations and results for each are shown below.
In addition to the wave equations and constants that are used elsewhere on this site in other applications, two important variables that are required to determine transverse energies and wavelengths are:
Distance – The distance between two particles, from the initial state to the final state. In the wave equations, this is expressed in terms of wavelengths (n) from the nucleus core to the electron.
Amplitude Difference – The energy released is also dependent on the amount of constructive or destructive wave interference between two particles. Amplitude factor (δ) is used in the wave equations to reflect this difference. A positron-electron interaction is set as the baseline of one, thus δ=1 for hydrogen energy calculations. However, other atomic elements will have different amplitude factors that will need to be considered. For this section, δ=1.
Hydrogen Ionization
When a particle experiences an amplitude difference, the transverse equation is modified to be the difference in energy from its initial position ni to its final position nf. In the case of ionization, an electron is ejected from an atom and experiences an amplitude difference, as it is no longer attracted to the atom’s nucleus. Once ejected, its distance (n wavelengths) is essentially infinity, so nf is set to infinity.
Using the Transverse Energy Equation, the final position is set to infinity, and the equation simplifies to:
A negative sign in this equation means that energy (a photon) needs to be absorbed. A positive value means that a photon is created.
A similar derivation can be used to calculate the photon’s wavelength required for ionization, starting with the Transverse Wavelength Equation and inserting infinity in place of nf.
Transverse Wavelength – Ionization
Using the orbital distances (n, in wavelengths) and amplitude factor of 1 (δ=1, for a single positron-electron interaction), the wave equations can now be used to calculate energies and wavelengths of particle interaction. Beginning with hydrogen, this example calculates the wavelength of a photon absorbed to eject an electron (K=10) from the first orbital (shell N1, or n=187,1789). Starting with the Transverse Wavelength – Ionization equation from above:
The equation above solves to be -9.113 x 10-8 meters, or –91.13 nanometers, a match of known hydrogen ionization energy data. In fact, the calculated values for each of the remaining orbitals (displayed in red in the table below) exactly match the known ionization energies and wavelengths of hydrogen data, shown in italics in the table.
In shell n=5, the ionization energy and wavelength of the electron-positron annihilation also match, showing a relation between ionization and annihilation energies as one equation; simply a difference of amplitude based on the distance between the particles.
Hydrogen Orbital Transitions
Another set of data demonstrating proof of the wave equations is the transitional energies and wavelengths for an electron in a hydrogen atom that moves between orbitals. In this case, it is a difference in energy between two positions relative to the nucleus, where ni is the initial orbital and nf is the final orbital.
For example, the wavelength distances for hydrogen for the third orbital (N3) is 1,690,098 wavelengths from the proton, and 751,155 wavelengths for the second orbital (N2). Thus for an electron that transitions from N=3 to N=2 (3->2) the wavelength values are: nf = 751,155 and ni = 1,690,098. Inserting these values into the Transverse Energy Interaction equation yields:
Note a positive value in the above energy calculation, meaning a photon is created. And using the Transverse Wavelength Interaction equation, the wavelength of this photon emitted for hydrogen orbital transition 3->2 is:
The above equation is solved to be 6.56 x 10-7 meters, or 656 nanometers. This is an exact match of photon wavelengths measured in experiments. The table below shows the calculations of the above transition (3->2) through to an electron in the ninth orbital transitioning to the second orbital (9->2). The calculations using the above equations are marked in red and the measured data from the hydrogen spectral series in italics.
CLICK TO ENLARGE
The above table is an exact match of the hydrogen spectrum, without the use of the Rydberg constant. It’s accurate to at least three digits and can be used not only for orbital transitions, but also for ionization energies and annihilation properties.
Hydrogen has one of the simplest emission spectra as there is only one electron per atom that can absorb and re-emit energy. |
The Hydrogen emission series
The electron in the ground state energy level of the hydrogen atom receives energy in the form of heat or electricity and is promoted to a higher energy level.
Ionization Energy Of Hydrogen Atom
It cannot remain at a higher level (excited state) for very long, and falls back to a lower level.
When the electron falls back down (relax) it must lose the energy difference between the two energy levels. This loss of energy is performed by releasing electromagnetic energy in the form of infrared, visible light or ultraviolet radiation.
Movement of electrons between the shells is called electron transitions.
When electron transitions take place the energy emitted can be detected and its wavelength measured. This provides information about the relative energies of the shells.
Ionisation Energy Of Hydrogen Atom Is 13.6
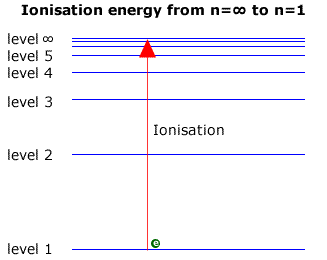
In the hydrogen atom (the simplest case with only one electron to 'jump' between shells) the energy emitted appears in several series of lines, each series corresponding to electrons falling back to different levels. This is shown in the diagram below.
The Lyman series corresponds to transitions between the higher shells and the lowest shell (ground state). The energy of these transitions produces radiation in the ultra-violet region of the spectrum
The energy shells are usually given a letter 'n' to describe the specific energy level. The lowest level is n=1, the second level is n=2 etc.

Transitions from higher shells (n>2) to n=2 produce radiation in the visible region of the spectrum. It can be seen by splitting the light using a prism or diffraction grating and projecting it onto a screen.
Convergence
As the energy levels increase in energy they get closer together. In other words level 2 and level 3 are further apart than level 3 and level 4. The levels converge towards a limit.
Transitions that occur in any series must also converge towards a high energy limit, as the largest transition is between the highest energy level and the level that is characteristic of the specific series.
The highest level is sometimes refered to as the 'infinite' level, as the levels get so close together where they converge that they are impossible to count.
Ionisation
When dealing with the Lyman series in the ultraviolet, the transitions are down to the ground state (level 1). The largest transition must represent a fall from the infinite level, ∞, to level 1. Viewed in reverse it can be considered to be equal to the ionisation energy, as this is the energy required to move an electron from the ground state to the infinite level (Note: Ionisation energy is usually expressed per mole of electrons).
| M(g) M+(g) + 1e |
Ionization Energy Of Hydrogen Atom
Consequently, the ionisation energy may be found by examining the Lyman series at the convergence limit. The wavelength of light corresponding to the convergence limit may be converted to energy using the relationship E = hc/l
Ionisation Energy Of Hydrogen Formula
Example: Calculate the ionisation energy of hydrogen, if the lines of the Lymann series converge to a limit at a wavelength of 90.1 nm. h, (Planck's constant) = 6.63 x 10-34, c, speed of light = 2.99 x 108 ms-1 E = hc/l wavelength 90.1 nm = 9.01 x 10-8 m Therefore Energy = (6.63 x 10-34 x 2.99 x 108)/9.01 x 10-8 ∴ E = 2.17 x 10-18 ∴ Energy/mole = 2.17 x 10-18 x 6.02 x 1023 ∴ Energy/mole = 1.308 x 106 Joules ∴ Energy = 1308 kJ mol-1 Ionisation energy of hydrogen = 1308 kJ mol-1 |
Determining Ionization Energy
This method may be used to find the first ionisation energy of any element.
Ionization Energy Of Hydrogen In Joules
The wavelength (or wavenumber) values corresponding to the convergence limits are available in data books or in the excellent NIST physics resource.
